License (open-access, http://creativecommons.org/licenses/by-sa/4.0/):
CC-BY-SA
License (open-access):
Prava korištenja: CC-BY-SA
License (open-access):
Journal content is published under CC-BY-SA licence.
Publication date: 2020
Volume: 19
Issue: 34
Recenzija, prikaz
What Are Map Projections and Why Are They So Called?
Miljenko Lapaine
The development of map projections ran in parallel with the production of maps and cartography in general. Over time, the development of many sciences, technical achievements, and the needs of everyday life initiated increasing demand for the production of various topographic and thematic maps of various scales and purposes, which required a continual increase in the number of map projections and the improvement of the mathematical basis of maps (Lapaine, Kuveždić 2007).
254055
17.10.2020.
![]()
Posjeta: 1.378 *
Birtić M, Blagus Bartolec G, Hudeček L, Jojić Lj, Kovačević B, Lewis K, Matas Ivanković I, Mihaljević M, Miloš I, Ramadanović E, Vidović D (2012) Školski rječnik hrvatskoga jezika. Institut za hrvatski jezik i jezikoslovlje i Školska knjiga,Zagreb. Također onlinehttps://hrcak.srce.hr/77954 ( 22.10.2020. )
HJP (2006) Hrvatski jezični portal, Znanje i Srce, Zagreb.http://hjp.znanje.hr ( 22.10.2020 )
Oxford English Dictionary , projecture prema francuskom projecture I latinskomhttps://www.lexico.com/definition/projecture ( 24.10.2020 )
Varenius B (1650) Mappas geographicas componere in his Geographia generalis, in qua affectiones generalis telluris explicantur...: Amsterdam, book 3, chap. 32, proposition 6, p. 691-725. [Latin. Construction of several existing projections described. Other editions at Amsterdam, 1664, 1671, 1672; Cambridge, 1672, 1681; Jena, 1693. Translated to English by Blome, Richard, 1682, as To compose geographical maps, in Cosmography and geography in two parts: The first ... being a translation from ... Varenius: London, p. 316-334. Translations also to English (1733), Dutch (1755), French (1755), Russian (1718, 1790).]
The development of map projections ran in parallel with the production of maps and cartography in general. Over time, the development of many sciences, technical achievements, and the needs of everyday life initiated increasing demand for the production of various topographic and thematic maps of various scales and purposes, which required a continual increase in the number of map projections and the improvement of the mathematical basis of maps (Lapaine, Kuveždić 2007).
The beginnings of map projection date back about two and a half thousand years, when Greek scientists first introduced mathematical principles into the foundations of mapping the Earth and heavens, and began to apply a network of meridians and parallels. The works of Anaximander, Eratosthenes, Thales, Apollonius and Hipparchus played a major role in the development of cartography.
It is believed that the first map in a map projection was made by Thales of Miletus in 600 BC. It was a map of the celestial sphere in the gnomonic projection. Among the oldest are stereographic and orthographic projections used by the famous Greek astronomer and mathematician Hipparchus, also for the purpose of mapping the celestial sphere, around 150 BC. From that time until today, several hundred different map projections have been invented.
The recently published Cartographic Dictionary (Frančula et al. 2020) contains this definition of map projection: “mapping a curved surface, especially an ellipsoid or sphere, into a plane”. The first reactions arrived a few days ago. One refers to the definition of map projection and reads as follows:
"Projection, map - you define it as mapping. It may have been necessary to take into account that mapping is a gerund that refers to an action (projecting), while the projection is the result of that action."
The name ‘map projection’ contains the word ‘projection’. Obviously the problem is in that word, which has a number of meanings, as is evident if we peek into any dictionary. According to Klaić'sLapaine 2017, the word ‘projection’ comes from the Latin projicere, of which the literal translation is ‘to throw before someone’, ‘to proffer’ (Klaić 1978).
If we look, for example, at the Croatian Language Portal (HJP 2006), we can find these definitions of the noun ‘projection’:
mat. A flattened image of a set of points in space obtained by projection, i.e. mapping, in which all the junctions of the associated pairs of points: a. either pass through one point [central projection] b. or are parallel to a given line [parallel projection; especially: orthogonal projection]
mat. An image of a set of points in a plane obtained by projecting (centrally or parallelly) onto a line in the same plane
film An enlarged photographic image transferred to the screen using an optical apparatus
fig. a. An idea or thought about something in the future [developmental projection] b. a prediction [projection of highway traffic]
psych. An unconscious defensive mechanism by which something emotionally unacceptable is unconsciously rejected and attributed (projected) to others
Apart from the fact that it is immediately obvious that ‘projection’ is not unambiguously defined, it seems that our critic was right, since according to the above, a projection is not mapping, but an image, i.e. the result of mapping. However, if we look at the School Dictionary of the Croatian Language (Birtić et al. 2017), we find this definition of the noun ‘projection’:
“noun. f. 1. mat. mapping the points of space onto a plane ◇ orthogonal ~ points on a line of operations by which a point is joined to the nearest point in a given direction; orthogonal ~ points on a plane of operations by which a point is joined to the nearest point on a given plane 2. An enlarged photographic image transferred by means of an optical device to a canvas ◇ cartographic ~ geo. the manner of transferring the spherical shapes of the Earth's surface onto a flat surface.”
This definition, in principle, is correct, except for these details: we distinguish between mapping onto a plane and mapping in a plane. Mapping onto a plane means that every point of the plane is an image of at least one point, which generally is not necessarily true. Therefore it would be better to say mapping in a plane. Furthermore, a map projection is often a mapping of the Earth’s sphere or ellipsoid in a plane, but it can also pertain to the Moon, Mars, or another planet or planetoid. Finally, geo. is an abbreviation for geography, and map projections are an integral part of cartography, which in Croatia, according to the official division of the sciences, is a branch of geodesy, not geography (OG 2009, 2012, 2013, 2016).
If we look at the Mathematical Dictionary (Gusić 1995), we find that projection is a term used in mathematics in various circumstances, but it is always a function that joins one point to another, or one vector to another. We differentiate central projection in space, central projection in a plane, orthogonal projection in space onto a plane, orthogonal projection in space onto a line, orthogonal projection in a plane onto a line, parallel projection, and so on. We have a very similar definition of projection in our Cartographic Dictionary.
From all this we can conclude that the term ‘map projection’ is rather awkward, as it can lead the uninitiated to the wrong conclusion that it is the result of projection, or mapping, rather than a special type of mapping that includes three notions: the area of definition or domain, mapping law, and the area of value or codomain (Lapaine 2017).
Let us now look at the term ‘map projection’ in some foreign languages:
It is easy to see that the root of the word ‘projection’ is present in the vast majority of these names.
Where did the name ‘projection’ for map projection come from?
One of the oldest books in which map projections are interpreted is Geographia generalis, in qua affectiones generalis telluris explicantur... by Bernhard Varenius, first published in 1664 in Amsterdam. This book is written in Latin, and Varenius uses the word projectura for projection. Richard Blome translated Varenius' book into English and published it in 1682 in London. He translated the Latin word projectura as projecture (from the French projecture and the classical Latin prōiect-, the past participle of prōicere + -ūra).
In Coup d'œil historique sur la projection des cartes de géographie,d’Avezac de Castera-Macaya (1863) explains the word ‘projection’: "D'abord le mot de projection lui-même aurait toute une histoire, s'il nous fallait raconter comment il fut emprunté au langage commun par la langue spéciale des géomètres, et comment par des applications successives, tantôt plus larges et tantôt plus restreintes, il est venu à signifier aujourd'hui, dans les opérations les plus usuelles de la géographie pratique, les divers modes de représentation à plat, sur le papier, de notre globe ou de quelqu'une de ses parties (1).
Cette opposition tranchée entre la forme sphérique de la terre et la surface plate sur laquelle il s'agit d'en transporter l'image, manifeste au premier coup d'oeil la difficulté radicale de la question, qui se refuse en effet à une solution rigoureusement exacte. Cependant, comme les méridiens et les parallèles terrestres partagent la surface convexe du sphéroïde en une multitude infinie de quadrilatères étagés par rangées depuis l'équateur jusqu'aux pôles (où ce sont des triangles qui forment la dernière rangée), et comme on peut, sans inconvénient sensible, considérer chacun de ces trapèzes élémentaires comme offrant une surface plane, le problème se résout en définitive à figurer sur le papier (ou sur tel autre plan qu'on aura choisi) des séries de quadrilatères se succédant et s'étageant entre eux d'une manière analogue à la disposition des facettes supposées du solide sphéroïdal; ou, en d'autres termes, à tracer à plat les lignes représentatives des méridiens et des parallèles terrestres, en un réseau (2) qui formera le canevas (3) de la carte.
Or, nous savons tous que pour effectuer ce tracé, l'on peut recourir à trois procédés divers de représentation: les vues perspectives, les développements de surfaces osculatrices, et les systèmes conventionnels. Nous en savons même tous bien davantage, car dans chacune de ces trois séries de problèmes indéterminés nous connaissons une quantité de solutions variées, quelques-unes parvenues à une sorte de vulgarité, d'autres au contraire restées en dehors de l'usage commun, toutes pourvues de dénominations plus ou moins exactes, destinées à caractériser chacune d'elles, quelquefois par son aspect matériel, mais plus régulièrement par ses propriétés spéciales, ou bien par le souvenir de son inventeur (ce qui n'est que justice), ou par celui du savant ingénieux qui en aura calculé et traduit en formules les lois mathématiques (ce qui n'est peut-être pas d'une aussi complète équité), ou encore par celui d'un simple vulgarisateur se substituant à l'auteur primitif (ce qui n'est plus équitable du tout).
Rien qu'à ces quelques mots, à mesure que je parle, je vois affluer sur vos lèvres tout un catalogue de dénominations et de noms propres, où sepressent, au premier rang, les projections stéréographique, centrale, orthographique, globulaire, plate, réduite, conique, homalographique, et les noms de Ptolémée, de Mercator, de Flamsteed, de La Hire, de Cassini, de Murdoch, de Lambert, d'Herschel., et ceux de Wright, d'Euler, de Lagrange, de Delambre, de Gauss et cent autres encore.
Oui, tous nous savonscela, etc est énorme! énorme. non sans doute le simple inventaire de la triple catégorie de projections décrites ou formulées par les adeptes, mais bien le bagage scientifique demeuré sousentendu, et qui se résume en ces projections.
Or quelle est, historiquement, la part des temps et des hommes, ou plutôt la part de chaque époque ou de chaque inventeur, dans cet ensemble de notions et de doctrines perfectionnées qu'il nous est permis de grouper aujourd'hui autour du mot projection? Nous avons ici, comme en. toute histoire, à percer l'obscurité des siècles pour y découvrir les plus anciens vestiges des essais tentés par les premiers pionniers qui ont ouvert la voie.
(1) Dans la rigueur onomastique, que l'usage n'a pas respertée, le mot de projection devait être réservé aux représentations directement obtenues d'après les lois de la perspective. Il a pu être étendu avec quelque apparence de raison aux développements qui supposaient l'existence d'une projection directe antérieure sur la surface développable; mais toute distinction a bientôt été perdue de vue, et le nom de projection s'applique vulgairement aujourd'hui à tout canevas de carte géographique. Cependant, si l'on voulait tenter de rétablir quelque exactitude dans la nomenclature relative à cet objet, c'est le mot de construction qu'il faudrait adopter comme appellation générale, comprenant, en trois catégories distinctes, les projections assujetties aux lois de la perspective, les développements subordonnés aux conditions de correlation de la sphère avec les surfaces développables, et les systèmes de représentation fondés sur des combinaisons purement conventionnelles.
(2) L'usage a plus particulièrement consacré le nom de réseau à l'assemblage des triangles déterminés sur le terrain par les grandes opérations géodésiques.
(3) Le mot de châssis est souvent employé aussi comme synonyme de canevas."
Translated into English, this reads: "First of all, the word projection itself would have a whole story of its own if we were to explain how it was borrowed from general language to the professional language of surveyors, and that by successive applications, sometimes wider and sometimes more limited, is used today in most common operations of practical geography, and denotes the different ways of depicting our globe or some of its parts, directly on paper (1).
The sharp contrast between the spherical shape of the Earth and the flat surface to which the image is to be transferred, at first glance, reveals the radical difficulty of the issue, which in fact rejects a strictly exact solution. However, while the Earth’s meridians and parallels divide the convex surface of spheroids into an infinite multitude of quadrilaterals proceeding in rows from the Equator to the poles (where the triangles form the last row), and as may be, without any noticeable defect, considering that each of these elementary trapezoids offers a flat surface, the problem is ultimately solved by presenting on paper (or another selected flat surface), a series of consecutive quadrilaterals in a manner analogous to the arrangement on a spheroidal body; or, in other words, drawing lines in a plane showing the network of terrestrial meridians and the parallels (2), that will form the foundation (3) of the map.
However, we all know that three different display methods can be used to produce such a drawing: perspective views, the development of oscillating surfaces, and conventional systems. We all know much more in fact, because in each of these three series of indeterminate problems we recognise many different solutions, some of which have reached a kind of triviality, while others, on the contrary, have remained out of common use; all have more or less exact names, whose purpose is to characterize each of them, sometimes by a material aspect, but more often by the particular properties or in memory of the inventor (which is fair), or of the genius scientist who calculates and translates mathematical laws into formulas (which may not be entirely fair), to that of the simple populariser who replaces the original author (which is no longer fair at all).

With these few words, as I speak, I see a whole catalogue of adjectives and proper names flowing to your lips, in which stereographic, central, orthographic, globular, flat, reduced, conic, and homolographic projections appear first, along with Ptolemy, Mercator, Flamsteed, La Hire, Cassini, Murdoch, Lambert, d'Herschel, and Wright, Euler, Lagrange, Delambre, Gauss, etc., and a hundred others.
Yes, we all know that “etc.” is huge! Huge. Undoubtedly, it is not a simple list of the three categories of projections described or formulated by followers, but the scientific baggage is still implied, and summarized in those projections.
However, what is historically a part of time and people, that is, a part of every epoch or every inventor, in the series of perfected notions and doctrines that we may today group around the word ‘projection’? Here, as in all of history, we need to break through the ambiguity of the centuries to reveal the oldest remnants of the attempts by which the first pioneers paved the way.
(1) In onomastic rigor, the use of which has not been respected, the word ‘projection’ should be reserved for representations obtained directly according to the laws of perspective. Perhaps the word ‘projection’ extended for some reason to developments which presupposed the existence of such a direct projection onto a surface that could be developed; but any difference was soon lost sight of, and the word ‘projection’ is today commonly applied to any geographical map. However, if we want to try to re-establish some accuracy in the nomenclature related to this subject, the word ‘construction’ should be accepted as a general term, containing in three different categories projections subject to the laws of perspective, developments subject to the correlation of spheres with surfaces that can be developed and displayed, and display systems based on purely conventional combinations.
(2) The use of the word ‘network’ is especially reserved for the assembly of triangles established in the field by major geodetic operations.
(3) The word ‘frame’ is also often used as a synonym for a map field.”
The second part of selected works byV. V. Kavrayskiy (1958) consists of three books in which he deals with the theory of map projections. In the first, General Theory of Map projections, he explains the word ‘projection’ in several places. So on pages 14-15 he writes, "В математике изобразить одну поверхность на другой значит установить взаимно однозначное соответствие между точками этих двух поверхностей. В интересующей нас области всякий математически определенный способ изображения всей или части поверхности земного эллипсоида или земного шара на плоскости носит название картографической проекции.
Закон, связывающий положение точки на поверхности земного шара или сфероида с положением изображения этой точки на плоскости - на карте, может быть выражен геометрически или же аналитически. Аналитическое выражение закона изображения достигается системой формул, позволяющих по координатам (например, широте и долготе) изображаемой точки вычислить координаты ее изображения (например, плоские прямоугольные или полярные).
В геометрии слово «проекция» обозначает либо ортогональную проекцию, в которой строяся простейцше технические чертежи, либо обладающую большей общностью перспективу.
В картографии же термин «проекция» имеет еще гораздо более общее значение. Не только перспектива земной поверхности, но и всякая математически определенная связь между положением точки в натуре и положением изображения этой точки на карте называется картографической проекцией. Большинство проекций, применяемых на практике для построения карт, как раз не являются перспективами."
Translated into English, this reads: "In mathematics, mapping one surface onto another means establishing correspondence between the points of the two surfaces. In our field of interest, any mathematically defined method of mapping all or part of the surface of the Earth's ellipsoid or Earth's sphere onto a plane is called a map projection.
The law that connects the position of a point on the surface of the Earth's sphere or spheroid with the position of the image of that point on the plane - on the map - can be expressed geometrically or analytically. The analytical expression of the mapping law is achieved by a system of formulas that allow the use of the coordinates (for example, the latitude and longitude) of the mapped point to calculate the coordinates of its image (for example, planar rectangular or polar).
In geometry, the word ‘projection’ means either an orthogonal projection, in which the simplest technical drawings are made, or a perspective that is more general.
In cartography, the term ‘projection’ has a much more general meaning. Map projection is not only the perspective of the Earth's surface, but also any mathematically determined relationship between the position of a point in nature and the position of the image of that point on the map. Most projections used to make maps in practice are not perspectives."
Conclusion
Map projections have been used for more than two millennia. They are mappings of curved surfaces, especially ellipsoids or spheres, transferred onto a plane, as stated in the Cartographic Dictionary (Frančula et al. 2020). The term ‘map projection’ entered cartography from mathematics, but it does not refer only to projections known in mathematics, such as perspective or central projection and axonometry, or parallel projection. The noun ‘projection’ in cartography should be understood conditionally, as it refers to any mapping of a curved surface, most commonly a sphere or ellipsoid, onto a plane.
Razvoj kartografskih projekcija tekao je usporedno s razvojem izradbe karata i kartografije općenito. Razvoj mnogih znanosti, tehnička dostignuća i potrebe svakodnevnoga života s vremenom su inicirali sve šire zahtjeve za izradbom raznovrsnih topografskih i tematskih karata različitog mjerila i namjene, što je zahtijevalo neprekidno povećavanje broja kartografskih projekcija i usavršavanje matematičke osnove karata (Lapaine, Kuveždić 2007).
Početci kartografskih projekcija stari su oko dvije i pol tisuće godina, otkad su grčki znanstvenici prvi uveli matematičke principe u temelje preslikavanja Zemlje i zvjezdanoga neba te počeli primjenjivati mrežu meridijana i paralela. Veliku su ulogu u razvoju kartografije odigrali radovi Anaksimandara, Eratostena, Talesa, Apolonija i Hiparha.
Smatra se da je prvu kartu u nekoj projekciji izradio Tales iz Mileta 600. godine pr. Kr. Bila je to karta nebeske sfere u gnomonskoj projekciji. Među najstarije se projekcije ubrajaju stereografska i ortografska koje je upotrijebio poznati grčki astronom i matematičar Hiparh, također za izradbu karata nebeske sfere oko 150. godine pr. Kr. Od toga doba do danas izumljeno je nekoliko stotina kartografskih projekcija.
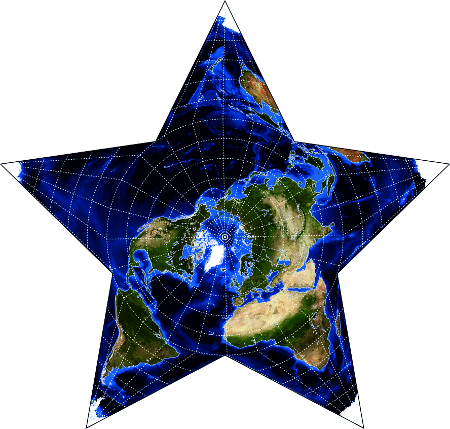
U nedavno objavljenom Kartografskom rječniku (Frančula i dr. 2020) nalazi se ova definicija kartografske projekcije: preslikavanje zakrivljene plohe, posebno elipsoida ili sfere, u ravninu. Prije nekoliko dana stigle su prve primjedbe. Jedna od njih odnosi se na definiciju kartografske projekcije i glasi ovako:
"Projekcija, kartografska - definirate ju kao preslikavanje… Možda je trebalo voditi računa o tome da je preslikavanje glagolska imenica koja se odnosi na radnju (projiciranje), a projekcija je rezultat te radnje."
Naša definicija kartografske projekcije istovjetna je definiciji iz mojeg članka objavljenog prije tri godine u rubrici Terminologija u časopisu Kartografija i geoinformacije (Lapaine 2017).
U nazivu kartografska projekcija nalazi se riječ projekcija. Očito je problem u toj riječi koja ima veći broj značenja u što se možemo lako uvjeriti ako zavirimo u bilo koji rječnik. Prema Klaićevu Rječniku stranih riječi riječ projekcija dolazi iz latinskoga projicere s doslovnim prijevodom baciti pred koga, pružiti (Klaić 1978).
Pogledamo li primjerice u Hrvatski jezični portal (HJP 2006) možemo naći ove definicije imenice projekcija:
mat. ravninska slika skupa točaka u prostoru dobivena projiciranjem, tj. preslikavanjem pri kojem sve spojnice pridruženih parova točaka: a. ili prolaze jednom točkom [centralna projekcija] b. ili su paralelne sa zadanim pravcem [paralelna projekcija; posebno: ortogonalna projekcija]
mat. slika nekog skupa točaka u ravnini dobivena projiciranjem (centralnim ili paralelnim) na pravac u istoj ravnini
film. na platno prenesena povećana slika fotografske snimke pomoću optičkog aparata
pren. a. ideja, zamisao o nečemu u budućnosti [projekcija razvoja] b. predviđanje [projekcija prometa na autocesti]
psih. nesvjesni obrambeni mehanizam kod kojega se ono što je emocionalno neprihvatljivo na nesvjestan način odbacuje i pripisuje (projicira) drugima
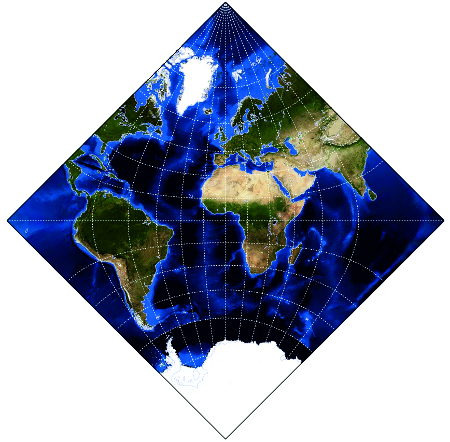
Osim što se odmah vidi da naziv projekcija nije jednoznačno definiran, čini se da je naš kritičar imao pravo jer prema navedenom projekcija nije preslikavanje, nego slika, tj. rezultat preslikavanja. Međutim, pogledamo li u Školski rječnik hrvatskoga jezika (Birtić i dr. 2017), možemo naći ovu definiciju imenice projekcija:
im. ž. 〈G projèkcijē; mn. N projèkcije, G projèkcījā〉 1. mat. preslikavanje točaka prostora na ravninu ◇ ortogonalna ~ točke na pravac operacija kojom se točki pridružuje najbliža točka na danome pravcu; ortogonalna ~ točke na ravninu operacija kojom se točki pridružuje najbliža točka na danoj ravnini 2. povećana slika fotografske snimke prenesena s pomoću optičkoga uređaja na platno ◇ kartografska ~ zem. način prenošenja sfernih oblika Zemljine površine na ravnu plohu
U toj je definiciji u načelu sve u redu, osim ovih detalja: razlikujemo preslikavanje na ravninu i u ravninu. Preslikavanje na ravninu značilo bi da je svaka točka ravnine slika bar jedne točke, što općenito ne mora biti. Dakle, bolje bi bilo da piše preslikavanje u ravninu. Nadalje, kartografska projekcija često je preslikavanje Zemljine sfere ili elipsoida u ravninu, ali može biti u igri Mjesec, Mars ili koji drugi planet ili planetoid. Konačno, zem. je kratica za zemljopis, a kartografske projekcije sastavni su dio kartografije koja je u Hrvatskoj prema službenoj podjeli znanosti grana geodezije, a ne geografije (NN 2009, 2012, 2013, 2016).
Pogledamo li u Matematički rječnik (Gusić 1995) naći ćemo da je projekcija pojam koji se u matematici koristi u raznim okolnostima, no uvijek je to funkcija koja jednoj točki pridružuje neku drugu točku ili vektoru vektor. Razlikuju se centralna projekcija u prostoru, centralna projekcija u ravnini, ortogonalna projekcija u prostoru na ravninu, ortogonalna projekcija u prostoru na pravac, ortogonalna projekcija u ravnini na pravac, paralelna projekcija itd. Vrlo sličnu definiciju projekcije imamo i u našem Kartografskom rječniku.
Iz svega rečenoga možemo zaključiti da je naziv kartografska projekcija malo nezgodan jer može neupućene navesti na pogrešan zaključak da je riječ o rezultatu projiciranja, odnosno preslikavanja, a ne o posebnoj vrsti preslikavanja koje uključuje tri pojma: područje definicije ili domenu, zakon preslikavanja i područje vrijednosti ili kodomenu (Lapaine 2017).
Pogledajmo sad kako se kartografska projekcija kaže na nekim stranim jezicima:
Lako se vidi da je u velikoj većini navedenih naziva prisutan korijen riječi projekcija.
Odakle naziv projekcija za kartografske projekcije?
Jedna od najstarijih knjiga u kojoj se tumače i kartografske projekcije je Geographia generalis, in qua affectiones generalis telluris explicantur...Bernharda Vareniusa, objavljena prvi put 1664. godine u Amsterdamu. Ta je knjiga napisana na latinskome jeziku, a Varenius za projekciju upotrebljava riječ projectura. Richard Blome preveo je na engleski i objavio Vareniusovu knjigu 1682. godine u Londonu. Latinsku riječ projectura preveo je s projecture prema francuskom projecture i klasičnom latinskom prōiect-, past particip od prōicere + -ūra..
U Povijesnom pogledu na projekciju geografskih karatad’Avezac de Castera-Macaya (1863) objašnjava riječ projekcija: "D'abord le mot de projection lui-même aurait toute une histoire, s'il nous fallait raconter comment il fut emprunté au langage commun par la langue spéciale des géomètres, et comment par des applications successives, tantôt plus larges et tantôt plus restreintes, il est venu à signifier aujourd'hui, dans les opérations les plus usuelles de la géographie pratique, les divers modes de représentation à plat, sur le papier, de notre globe ou de quelqu'une de ses parties (1).
Cette opposition tranchée entre la forme sphérique de la terre et la surface plate sur laquelle il s'agit d'en transporter l'image, manifeste au premier coup d'oeil la difficulté radicale de la question, qui se refuse en effet à une solution rigoureusement exacte. Cependant, comme les méridiens et les parallèles terrestres partagent la surface convexe du sphéroïde en une multitude infinie de quadrilatères étagés par rangées depuis l'équateur jusqu'aux pôles (où ce sont des triangles qui forment la dernière rangée), et comme on peut, sans inconvénient sensible, considérer chacun de ces trapèzes élémentaires comme offrant une surface plane, le problème se résout en définitive à figurer sur le papier (ou sur tel autre plan qu'on aura choisi) des séries de quadrilatères se succédant et s'étageant entre eux d'une manière analogue à la disposition des facettes supposées du solide sphéroïdal; ou, en d'autres termes, à tracer à plat les lignes représentatives des méridiens et des parallèles terrestres, en un réseau (2) qui formera le canevas (3) de la carte.
Or, nous savons tous que pour effectuer ce tracé, l'on peut recourir à trois procédés divers de représentation : les vues perspectives, les développements de surfaces osculatrices, et les systèmes conventionnels. Nous en savons même tous bien davantage, car dans chacune de ces trois séries de problèmes indéterminés nous connaissons une quantité de solutions variées, quelques-unes parvenues à une sorte de vulgarité, d'autres au contraire restées en dehors de l'usage commun, toutes pourvues de dénominations plus ou moins exactes, destinées à caractériser chacune d'elles, quelquefois par son aspect matériel, mais plus régulièrement par ses propriétés spéciales, ou bien par le souvenir de son inventeur (ce qui n'est que justice), ou par celui du savant ingénieux qui en aura calculé et traduit en formules les lois mathématiques (ce qui n'est peut-être pas d'une aussi complète équité), ou encore par celui d'un simple vulgarisateur se substituant à l'auteur primitif (ce qui n'est plus équitable du tout).
Rien qu'à ces quelques mots, à mesure que je parle, je vois affluer sur vos lèvres tout un catalogue de dénominations et de noms propres, où sepressent, au premier rang, les projections stéréographique, centrale, orthographique, globulaire, plate, réduite, conique, homalographique, et les noms de Ptolémée, de Mercator, de Flamsteed, de La Hire, de Cassini, de Murdoch, de Lambert, d'Herschel., et ceux de Wright, d'Euler, de Lagrange, de Delambre, de Gauss et cent autres encore.
Oui, tous nous savonscela, etc est énorme! énorme. non sans doute le simple inventaire de la triple catégorie de projections décrites ou formulées par les adeptes, mais bien le bagage scientifique demeuré sousentendu, et qui se résume en ces projections.
Or quelle est, historiquement, la part des temps et des hommes, ou plutôt la part de chaque époque ou de chaque inventeur, dans cet ensemble de notions et de doctrines perfectionnées qu'il nous est permis de grouper aujourd'hui autour du mot projection? Nous avons ici, comme en. toute histoire, à percer l'obscurité des siècles pour y découvrir les plus anciens vestiges des essais tentés par les premiers pionniers qui ont ouvert la voie.
(1) Dans la rigueur onomastique, que l'usage n'a pas respertée, le mot de projection devait être réservé aux représentations directement obtenues d'après les lois de la perspective. Il a pu être étendu avec quelque apparence de raison aux développements qui supposaient l'existence d'une projection directe antérieure sur la surface développable; mais toute distinction a bientôt été perdue de vue, et le nom de projection s'applique vulgairement aujourd'hui à tout canevas de carte géographique. Cependant, si l'on voulait tenter de rétablir quelque exactitude dans la nomenclature relative à cet objet, c'est le mot de construction qu'il faudrait adopter comme appellation générale, comprenant, en trois catégories distinctes, les projections assujetties aux lois de la perspective, les développements subordonnés aux conditions de correlation de la sphère avec les surfaces développables, et les systèmes de représentation fondés sur des combinaisons purement conventionnelles.
(2) L'usage a plus particulièrement consacré le nom de réseau à l'assemblage des triangles déterminés sur le terrain par les grandes opérations géodésiques.
(3) Le mot de châssis est souvent employé aussi comme synonyme de canevas."
U prijevodu na hrvatski jezik taj citat glasi: "Prije svega, sama riječ projekcija imala bi cijelu pripovijest kad bismo morali reći kako ju je iz zajedničkog jezika posudio poseban jezik geometara, a kako je uzastopnim primjenama, ponekad širim, a ponekad i ograničenijim, danas u najčešćim operacijama praktične geografije i označava različite načine prikazivanja našeg globusa ili nekih njegovih dijelova ravno, na papiru, (1).
Oštra suprotnost između sfernog oblika Zemlje i ravne površine na koju treba prenijeti sliku, na prvi pogled očituje radikalnu poteškoću pitanja, koje zapravo odbija strogo egzaktno rješenje. Međutim, dok Zemljini meridijani i paralele dijele konveksnu površinu sferoida u beskonačnom mnoštvu četverokuta stupajući u redovima od ekvatora do polova (gdje trokuti čine posljednji red), i kao što može biti, bez ikakvog primjetnog nedostatka, uzimajući u obzir da svaki od tih elementarnih trapeza nudi ravnu površinu, problem je u konačnici riješen da se pojavi na papiru (ili na nekoj drugoj odabranoj ravnoj plohi) niz uzastopnih četverokuta na način analogan rasporedu na sferoidnome tijelu; ili, drugim riječima, povući u ravnini linije koje će prikazivati mrežu zemaljskih meridijana i paralela (2) koja će oblikovati temelj (3) karte.
Međutim, svi znamo da se za izvođenje takvog crtanja može pribjeći trima različitim metodama prikaza: perspektivnim pogledima, razvoju oskulirajućih ploha i konvencionalnim sustavima. Svi čak znamo i mnogo više, jer u svakoj od te tri serije neodređenih problema poznajemo mnoštvo različitih rješenja, neka su dosegla neku vrstu trivijalnosti, a druga su, naprotiv, ostala izvan uobičajene upotrebe, sva imaju više ili manje egzaktna imena, čija je svrha karakterizirati svako od njih, ponekad i materijalnim aspektom, ali češće posebnim svojstvima ili sjećanjem na izumitelja (što je pravedno), ili onim genijalnog znanstvenika koji će izračunati i prevesti matematičke zakone u formule (što možda nije baš potpuno pravedno) ili onim jednostavnog popularizatora koji zamjenjuje izvornog autora (što više uopće nije pošteno).

Upravo s ovih nekoliko riječi, dok govorim, vidim čitav katalog naziva i vlastitih imena kako vam teku prema usnama, u kojima se u prvom redu pojavljuju stereografske, centralne, ortografske, globularne, ravne, reducirane, konusne, homalografske projekcije i imena Ptolomeja, Mercatora, Flamsteeda, La Hirea, Cassinija, Murdocha, Lamberta, d'Herschela i imena Wrighta, Eulera, Lagrangea, Delambrea, Gaussa itd. još stotinu drugih.
Da, svi znamo da je itd. ogromno! Ogromno. Nesumnjivo nije jednostavan popis triju kategorija projekcija koje su opisali ili formulirali sljedbenici, ali znanstvena prtljaga je i dalje bila podrazumijevana, a sažeta je u tim projekcijama.
Međutim, što je, povijesno, dio vremena i ljudi, odnosno dio svake epohe ili svakog izumitelja, u tom nizu usavršenih predodžbi i doktrina koje danas smijemo grupirati oko riječi projekcija? Trebamo ovdje, kao u cijeloj povijesti, probiti nejasnoću stoljeća kako bismo otkrili najstarije ostatke pokušaja kojima su prvi pioniri utrli put.
(1) U onomastičkoj strogosti, koju upotreba nije poštivala, riječ projekcija trebala bi biti rezervirana za prikaze izravno dobivene prema zakonima perspektive. Možda se riječ projekcija proširila s nekim razlogom na razvoje koji su pretpostavljali postojanje navedene izravne projekcije na plohu koja se može razviti; ali se svaka razlika ubrzo izgubila iz vida, a naziv projekcije danas se uobičajeno primjenjuje na bilo koju geografsku kartu. Međutim, ako bismo željeli pokušati ponovno uspostaviti određenu točnost u nomenklaturi koja se odnosi na taj predmet, riječ konstrukcija trebala bi biti prihvaćena kao opći naziv, koji sadrži, u tri različite kategorije, projekcije podložne zakonima perspektive, razvoje podređene uvjetima korelacije sfere s plohama koje se mogu razvijati i sustave prikazivanja utemeljene na čisto konvencionalnim kombinacijama.
(2) Upotreba je naziv mreža posebno posvetila sastavljanju trokuta utvrđenih na terenu velikim geodetskim operacijama.
(3) Riječ okvir također se često koristi kao sinonim za polje karte."
Drugi dio izabranih radovaV. V. Kavrajskoga (1958) sastoji se od tri knjige u kojima se bavi teorijom kartografskih projekcija. U prvoj od njih pod naslovom Opća teorija kartografskih projekcija na nekoliko mjesta objašnjava naziv projekcija. Tako na str. 14-15 možemo pročitati ovo: "В математике изобразить одну поверхность на другой значит установить взаимно однозначное соответствие между точками этих двух поверхностей. В интересующей нас области всякий математически определенный способ изображения всей или части поверхности земного эллипсоида или земного шара на плоскости носит название картографической проекции.
Закон, связывающий положение точки на поверхности земного шара или сфероида с положением изображения этой точки на плоскости - на карте, может быть выражен геометрически или же аналитически. Аналитическое выражение закона изображения достигается системой формул, позволяющих по координатам (например, широте и долготе) изображаемой точки вычислить координаты ее изображения (например, плоские прямоугольные или полярные).
В геометрии слово «проекция» обозначает либо ортогональную проекцию, в которой строяся простейцше технические чертежи, либо обладающую большей общностью перспективу.
В картографии же термин «проекция» имеет еще гораздо более общее значение. Не только перспектива земной поверхности, но и всякая математически определенная связь между положением точки в натуре и положением изображения этой точки на карте называется картографической проекцией. Большинство проекций, применяемых на практике для построения карт, как раз не являются перспективами."
U prijevodu na hrvatski jezik taj citat glasi: "U matematici preslikati jednu plohu na drugu znači uspostaviti međusobnu korespondenciju između točaka tih dviju ploha. U području koje nas zanima, bilo koja matematički definirana metoda preslikavanja cijele ili dijela površine Zemljina elipsoida ili Zemljine sfere u ravninu naziva se kartografskom projekcijom.
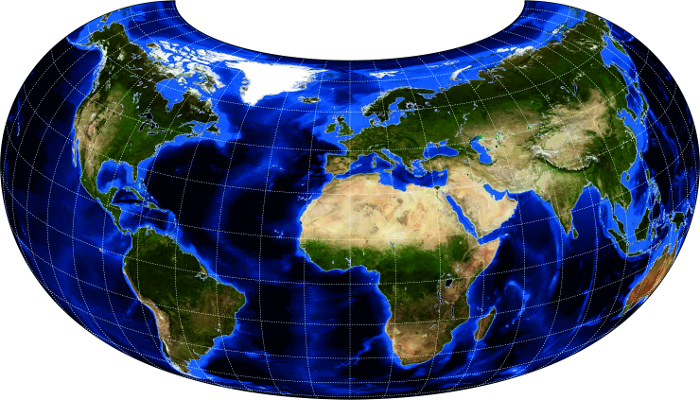
Zakon koji povezuje položaj točke na plohi Zemljine kugle ili sferoida s položajem slike te točke u ravnini – na karti, može se izraziti geometrijski ili analitički. Analitički izraz zakona preslikavanja postiže se sustavom formula koje omogućuju upotrebu koordinata (na primjer, geografske širine i dužine) preslikavane točke za računanje koordinata njezine slike (na primjer, ravninske pravokutne ili polarne).
U geometriji riječ "projekcija" označava ili ortogonalnu projekciju, u kojoj se izrađuju najjednostavniji tehnički crteži, ili perspektivu koja je općenitija.
U kartografiji naziv "projekcija" ima puno općenitije značenje. Kartografskom projekcijom naziva se ne samo perspektiva Zemljine površine, već i svaki matematički određeni odnos između položaja točke u prirodi i položaja slike te točke na karti. Većina projekcija koje se u praksi koriste za izradu karata nisu perspektive."
Zaključak
Kartografske projekcije koriste se više od dva tisućljeća. To su preslikavanja zakrivljene plohe, posebno elipsoida ili sfere, u ravninu kako stoji u Kartografskom rječniku (Frančula i dr. 2020). Naziv kartografske projekcije ušao je u kartografiju iz matematike, ali se ne odnosi samo na projekcije poznate u matematici kao primjerice perspektiva ili centralna projekcija i aksonometrija ili paralelno projiciranje. Imenicu projekcija u kartografiji treba shvatiti uvjetno jer se odnosi na bilo koje preslikavanje zakrivljene plohe, najčešće sfere ili elipsoida, u ravninu.